All Insights
Podcasts
Podcast: Harnessing the Storm: Data-Driven Decision Making with Glen McCracken
Articles

Data should provide your questions, not just your answers
Articles
Diving into the deep blue data lake, maths in 3072 dimensions, and the perfect recipe for Data Spaghetti
Articles

What’s up with all these “Data-X” words?
Podcasts
Data as an Asset: Navigating the Future with BDO’s Chief Data Officer, Denholm Hesse
Articles
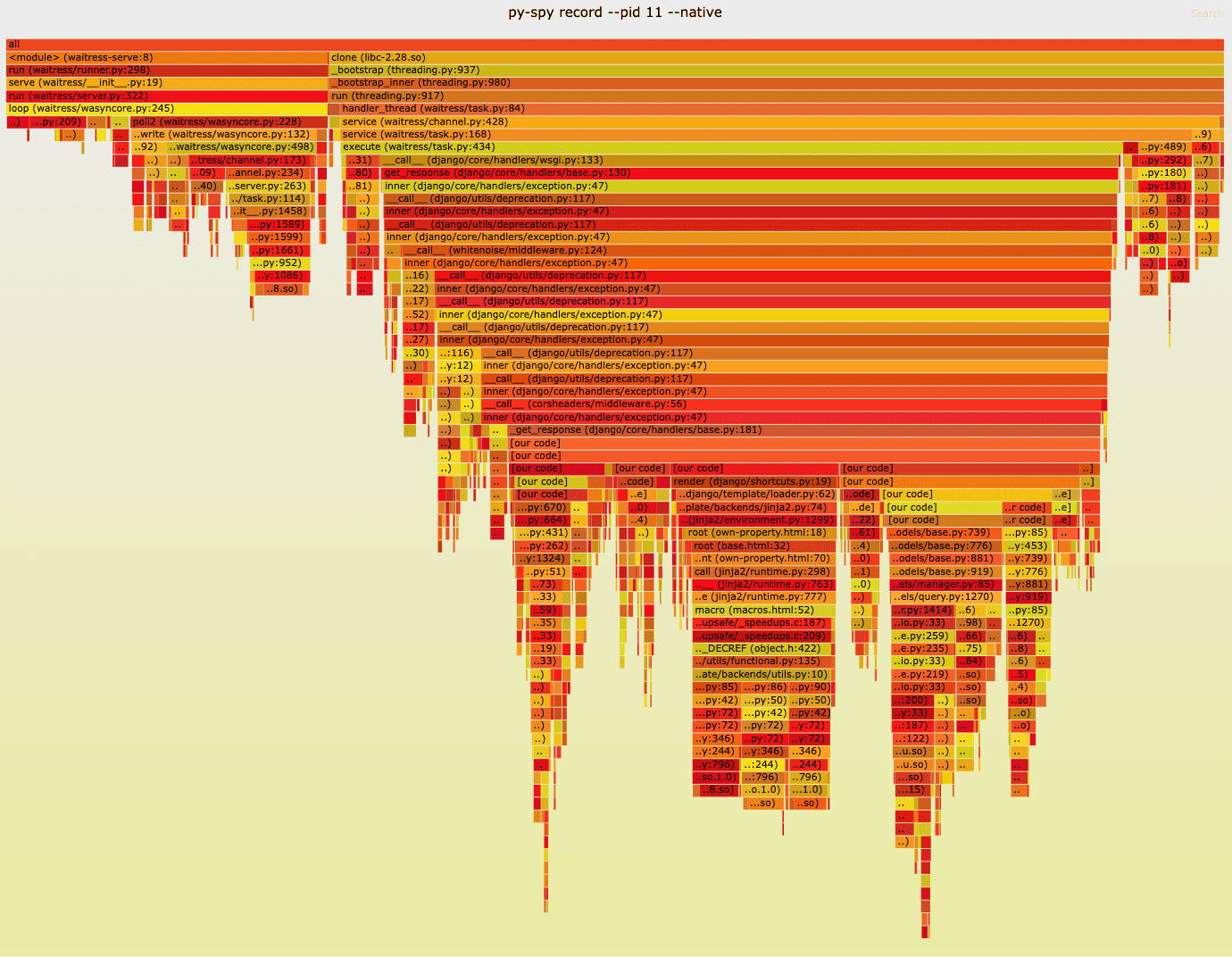
A love letter to flame graphs: or why the perfect visualisation makes the problem obvious
Articles
Showing up as a real human person is the only way to be an effective mentor and manager
Articles

Women in tech only want one thing and it’s…
Articles

Six senior Softwire women on careers, self-belief and success
Podcasts
Podcast: Empowering Innovation: Women Who Code with Rajani Rao
Podcasts
Podcast: Thomas Harris on navigating digital transformation: Insights from MasterCard’s journey
Podcasts